A Report on Vocational Training in Chicago and in Other Cities
Chapter 15: The Test in Arithmetic
Table of Contents | Next | Previous
The first part of the test in arithmetic is a set of four problems, which we will term the " fundamentals," taken from the list used by J.N. Rice in his extensive tests of school children, the results of which were published in the Forum, Volume 34. The first problem is of fourth-grade difficulty, the second and third should be worked in the fifth grade, the fourth because of the decimal would be classified as a sixth-grade problem. The last part of the arithmetic test is a group of four problems which might be called an exercise in objectified fractions. which teachers assure me would be easy for properly instructed fifth-grade pupils.
The arithmetic test was made uniform for all, except in the case of a few boys who had left school, below the sixth grade. They were given a somewhat easier test.
The two sets of problems given to all above the fifth grade are herewith appended.
Set I. Fundamentals
1. If a boy pays $2.83 for 100 papers, and sells them at 4 cents apiece, how much does he make:
2. A flour merchant bought 1,437 barrels of flour at $7 a barrel. He sold 900 of these barrels at $9 a barrel and the remainder at $6 a barrel. How much did he make?
3. If a train runs 31 2/3 miles an hour, how long will it take a train to run from Buffalo to Omaha, a distance of 1,045 miles?
4. A farmer's wife bought 2.75 yards of table linen at 87 cents a yard and 16 yards of flannel at 55 cents a yard. She paid in butter at 27 cents a pound. How many pounds of butter was she obliged to give?
(279)
1. On the line X Y mark off a length which is j of y, of the whole length X Y [use a ruler].
![]()
2. On the figure A B C D mark off an area (surface) which is 1/3 of 1/2 of
the whole area A B C D [use a ruler].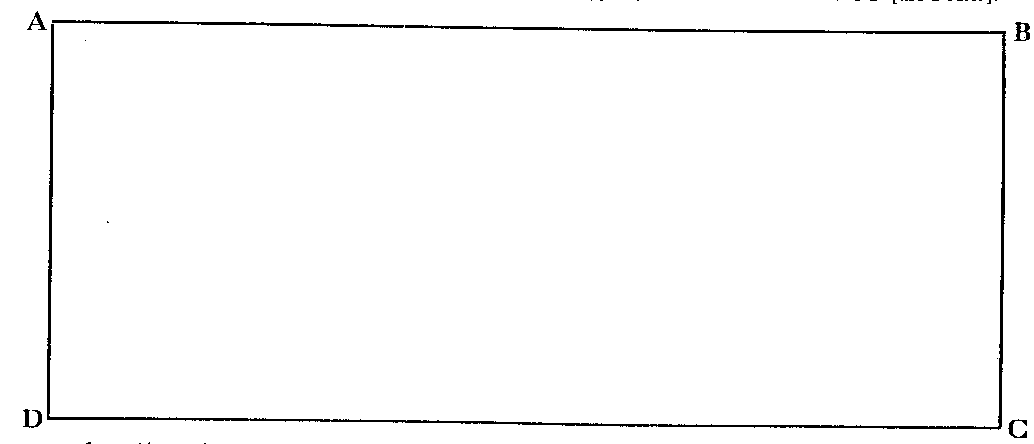
3. In problem 2, how many square inches are there in the area marked off?
4. How many square feet?
280)
The first two problems involve only simple operations in the fundamental processes of multiplication, subtraction and addition. The third problem calls for knowledge of fractions and division. while the fourth contains a decimal.
The second set involves nothing but fractions put in practical form. Problems involving square root, per cent or interest, depending more or less on the memory of a rule for their solution, were intentionally left out.
It seems fair to say that this test represents the minimum amount of arithmetic that any reasonably equipped child should carry from school into life.
The method of grading was as follows:
(a) A problem solved correctly in all particulars we called -- Right.
(b) Where mechanical error only occurred we graded the answer as correct in -- Principle.
(c) The third ranking was -- Wrong.
(d) The fourth- Not attempted.
We feel that this form shows the essential facts better than would any device for indicating percentages.
Separate tables were made for each problem showing relative standing of each grade on each of the eight problems.
The papers and tables show the following significant facts:
(a) In the three groups of boys on a test which should presumably be equally easy for all, the ranks range consistently downward for each grade from high school to sixth.
Group I (Night school)
The totals for this group (as show in Table V) reveal that of the boys of the eighth grade 76 per cent solve correctly both as to method and mechanical execution all the problems in the test on fundamentals. In like manner, 60 per cent solve correctly all the problems in the rectangle test.
Of the seventh-grade boys of Group I 56 per cent solve the fundamental tests and 42 per cent the rectangle tests.
In the sixth grade, Group I, the percentages are 36 per cent correct for the fundamental and 34 per cent correct for the rectangle problems.
(281)
Group II (Apprentice Schools)
Table V reveals that of high-school boys (none of which grade appear in the preceding group) 96 per cent solve correctly both as to method and mechanical accuracy all the problems in the fundamental tests. 92 per cent of the rectangle tests.
For the 8th grade the percentages are 88 per cent and 86 per cent.
For the 7th grade 76 per cent and 67 per cent.
For the 6th grade 66 per cent and 31 per cent.
Group III (Boys out of school)
High school, 79 per cent on fundamentals, 86 per cent on the rectangle
tests.
Eighth grade, 62 per cent and 54 per cent.
Seventh grade, 48 per cent and 23 per cent.
Sixth grade, 36 per cent and 22 per cent.
Table six shows the totals for each grade throughout the three groups.
High school- fundamentals 88 per cent, rectangle 89 per cent.
Eighth grade- fundamentals 75 per cent, rectangle 67 per cent.
Seventh grade-fundamentals 60 per cent, rectangle 42 per cent.
Sixth grade - fundamentals 46 per cent, rectangle 29 per cent.
(b) The difference in favor of the higher grades is not always in knowledge of the principles involved but in speed, accuracy and neatness - qualities that are particularly developed in the schoolroom. This is shown by the papers themselves.
When the sixth-grade boy gets the principle of his problem wrong he becomes so involved that he also fails in mechanical accuracy, while an eighth-grade boy, even if he err in the principle of his problem, will often carry it out correctly in all other respects. This fact largely accounts for the difference between the higher and lower grades in the solution of the first or easiest problem in fundamentals. This difference is:
Group I. 8th grade, 91 per cent; 7th, 77 per cent; 6th, 63 per cent.
Group II. 8th grade, 98 per cent; 7th, 97 per cent; 6th, 86 per cent.
Group III. 8th grade, 90 per cent; 7th, 87 per cent; 6th, 73 per cent.
282) (c) That the difference between the rankings of the grades is not merely due to a difference in degree of mechanical skill, but also involves knowledge of principles is shown by the discrepancy between the higher and lower grades in the solution of the fourth, the most difficult of the fundamental problems. The following figures reveal this:
Group I. 8th grade, 58 per cent; 7th. 32 per cent; 6th, 10 per cent.
Group II. 8th grade, 80 per cent; 7th, 61 per cent; 6th, 38 per cent.
Group III. 8th grade, 36 per cent; 7th, 19 per cent; 6th, 0 per cent.
(d) This difference extends to the ability to reason-to handle the real problems that come before men in actual life. That the boy who leaves school in the sixth grade is at a distinct disadvantage in comparison with the one who remains longer in school is shown by the totals in table six for the work on the rectangle: 8th grade, 67% per cent, 7th, 42 per cent, 6th, 29 per cent. This is more strikingly illustrated in the details found in Table V, especially in Group III.
Group I. 8th grade, 60 per cent; 7th, 42 per cent; 6th, 34 per cent.
Group II. 8th grade, 86 per cent; 7th, 67 per cent; 6th, 31 per cent.
Group III. 8th grade, 54 per cent; 7th, 23 per cent; 6th, 22 per cent.
The discrepancy between grades in ability to solve these problems is not due to a difference in age. As already noted, the average age of the boys from the sixth is somewhat greater than that of those in the grades above them. Is the lower standing of the lower grades due in part to lack of school training? Further discussion will throw light on this question.
We now undertake to prove from our tables that the discrepancy in the intellectual powers of the boy who leaves school at the sixth grade, and that of the one who remains through the seventh, eighth, or even beyond, is not merely due to selection-that is, it is not due simply to the fact that a dull boy is more likely to leave school in the lower grades than is his brighter schoolmate. The difference is due in part to the actual difference in school training. Our tables distinctly show that the boy who leaves school at the sixth grade, and
(283) then attends the night school or the Apprentice Schools, tends to improve his intellectual powers above that of the one who remains out of school. To prove this, note the relative standing of the different grades in the three groups.
In the eighth grade, 12 per cent more boys of the Apprentice School solve the fundamentals rightly than did those of the night schools who are not getting as much drill in arithmetic. In the rectangle problems this difference is 26 per cent in favor of the apprentices. In the sixth grade the difference between Groups I and II is 30 per cent in favor of the Apprentice Schools. In the rectangle problems for some reason that is not clear the difference is the other way, 3 per cent.
The difference between the eighth grade of the night school and the eighth grade of those out of school is 14 per cent in favor of the former in the fundamentals. In the rectangle the difference is 6 per cent in favor of the night schools. In the sixth grade, between the night schools and those out of school no difference exists. In the rectangle the difference is 12 per cent in favor of the night schools.
Assuming, then, that the same type of boys quit school at a given grade, the one who attends the night school or the Apprentice Schools gains over the one who does not. Is there a natural selection here? The testimony of the teachers is to the contrary.
The tables show that even in the eighth grade a considerable number of the boys fail to solve these fifth and sixth grade problems. Other tables exists which show that a larger per cent of students in the corresponding grades. who are attending day school, solve problems of equal difficulty. This would seem to show an actual total loss to the boy who leaves school before completing the eighth grade.The arithmetic tests. Table V, reveal a marked superiority on the part of the boys of the Apprentice Schools over those of the night schools, and over those out of school, the difference often being as great as 30 per cent. This would seem to be due to the direct correlation of the work in arithmetic in the Apprentice Schools with the trade the boys are learning. In other subjects, such as English, the apprentices were by no means superior to the other boys.
284) Eighteen boys of the fifth grade, nine of the fourth and one of the third were tested. For them, the two least difficult problems that were used for tile testing of the higher grade boys, and two yet more simple were used. The problems follow
1. What will 24 quarts of cream cost at $1.20 a gallon?
2. If a boy pays $2.83 for 100 papers and sells them at 4 cents apiece, how much does he make?
3. If I buy 8 dozen pencils at 37 cents a dozen and sell them at 5 cents apiece, how much do I make?
4. A flour merchant bought 1,437 barrels of flour at $7 a barrel. He sold 800 of these barrels at $9 a barrel, and the remainder at $6 a barrel. How much did he make?
This test is certainly not more difficult than those employed for pupils of the fourth grade of the public schools. The rectangle tests were also given to these twenty-eight boys. Reference to Tables It' and IV-A will reveal the pitiful inability of a large majority of these boys to solve even the most simple of these problems.
The total number of these boys tested in arithmetic was. in fundamentals, 655, and in the rectangle 610.
(285)
| Problem 1 | Problem 2 | Problem 3 | Problem 4 | Total % | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Gr. | No. | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt. | Pr. |
| 8 | 111 | 101 | 91 | 2 | 2 | 87 | 78 | 15 | 14 | 48 | 76 | 10 | 9 | 64 | 58 | 13 | 12 | 76 | 9 |
| 7 | 111 | 86 | 77 | 3 | 3 | 71 | 64 | 17 | 15 | 45 | 51 | 12 | 11 | 38 | 34 | 16 | 14 | 56 | 11 |
| 6 | 86 | 54 | 63 | - | - | 35 | 42 | 6 | 7 | 23 | 27 | 11 | 13 | 16 | 12 | 4 | 5 | 36 | 6 |
| Gr. | No. | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W.. | N. Att |
| 8 | 111 | 8 | 7 | - | - | 9 | 8 | - | - | 14 | 13 | 3 | 3 | 32 | 29 | 2 | 2 | 16 | 1 |
| 7 | 111 | 22 | 20 | - | - | 23 | 21 | 1 | 1 | 30 | 27 | 11 | 10 | 43 | 39 | 14 | 13 | 27 | 6 |
| 6 | 86 | 32 | 37 | - | - | 44 | 51 | 1 | 1 | 32 | 40 | 18 | 20 | 44 | 51 | 28 | 31 | 47 | 13 |
The above table shows the ranking of the night school in each of the
four fundamental problems. (Set I). Reading from left to right are
shown the grade, the number in the grade, the number who worked the
first problem correctly, the per cent who worked the first problem
correctly, the number who had the principle right, but made some
mechanical error, and the per cent, and so on, for the four problems.
At the end is shown the total right per cent and the total principle
right per cent.
The lower half of the table shows the number who got the first problem wrong, the per cent, the number who did not attempt it, and the per cent, etc.
(286)
| Problem 1 | Problem 2 | Problem 3 | Problem 4 | Total % | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Gr. | No. | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt. | Pr. |
| 8 | 100 | 87 | 87 | - | - | 61 | 61 | - | - | 53 | 53 | - | - | 38 | 38 | - | - | 60 | - |
| 7 | 98 | 73 | 72 | - | - | 49 | 50 | - | - | 31 | 32 | - | - | 15 | 15 | - | - | 42 | - |
| 6 | 72 | 47 | 65 | - | - | 25 | 35 | - | - | 19 | 26 | - | - | 8 | 11 | - | - | 34 | - |
| Gr. | No. | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W.. | N. Att |
| 8 | 100 | 13 | 13 | - | - | 37 | 37 | 2 | 2 | 34 | 34 | 3 | 3 | 34 | 34 | 28 | 28 | 30 | 8 |
| 7 | 98 | 23 | 24 | 2 | 2 | 44 | 45 | 5 | 5 | 38 | 39 | 29 | 30 | 42 | 43 | 41 | 42 | 38 | 22 |
| 6 | 72 | 22 | 31 | 3 | 4 | 39 | 54 | 8 | 11 | 22 | 31 | 31 | 44 | 24 | 33 | 40 | 56 | 37 | 29 |
The above table is arranged in the same manner as Table I and records the results of the night school in the rectangle problem
(287)
| Problem 1 | Problem 2 | Problem 3 | Problem 4 | Total % | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Gr. | No. | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt. | Pr. |
| HS | 6 | 6 | 100 | - | - | 6 | 100 | - | - | 6 | 100 | - | - | 5 | 83 | 1 | 17 | 96 | - |
| 8 | 82 | 80 | 98 | 1 | 1 | 69 | 80 | 10 | 12 | 76 | 93 | 3 | 4 | 60 | 90 | 10 | 12 | 88 | - |
| 7 | 33 | 32 | 97 | 1 | 3 | 23 | 70 | 8 | 24 | 25 | 76 | 2 | 6 | 20 | 61 | 1 | 3 | 73 | - |
| 6 | 21 | 18 | 86 | - | - | 15 | 71 | 4 | 19 | 14 | 67 | 3 | 14 | 8 | 38 | 2 | 10 | 66 | - |
| Gr. | No. | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W.. | N. Att |
| HS | 6 | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - |
| 8 | 82 | 1 | 1 | - | - | 3 | 4 | - | - | 3 | 4 | - | - | 6 | 7 | - | - | 7 | - |
| 7 | 33 | - | - | - | - | 2 | 6 | - | - | 6 | 18 | - | - | 11 | 33 | 1 | 3 | 14 | 1 |
| 6 | 21 | 3 | 14 | - | - | 1 | 5 | 1 | 5 | 1 | 5 | 3 | 14 | 7 | 33 | 4 | 19 | 14 | 10 |
The results of the problems for the Apprentice Schools in the fundamentals (Set I)
(288)
| Problem 1 | Problem 2 | Problem 3 | Problem 4 | Total % | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Gr. | No. | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt. | Pr. |
| HS | 6 | 6 | 100 | - | - | 6 | 100 | - | - | 5 | 83 | - | - | 5 | 83 | - | - | 92 | |
| 8 | 81 | 78 | 96 | - | - | 74 | 91 | - | - | 66 | 81 | - | - | 61 | 75 | - | - | 86 | |
| 7 | 35 | 27 | 77 | - | - | 26 | 74 | - | - | 22 | 62 | - | - | 12 | 34 | - | - | 62 | |
| 6 | 22 | 14 | 63 | - | - | 9 | 40 | - | - | 4 | 18 | - | - | 1 | 4 | - | - | 31 | |
| Gr. | No. | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W. | N. Att |
| HS | 6 | - | - | - | - | - | - | - | - | 12 | 16 | - | - | 1 | 16 | - | - | 8 | - |
| 8 | 81 | 1 | 1 | 3 | 2 | 5 | 6 | 2 | 2 | 8 | 15 | 3 | 4 | 16 | 20 | 4 | 5 | 11 | 3 |
| 7 | 35 | 3 | 9 | 5 | 14 | 5 | 14 | 4 | 11 | 14 | 23 | 5 | 14 | 18 | 51 | 5 | 14 | 26 | 13 |
| 6 | 22 | 6 | 27 | 2 | 9 | 10 | 45 | 3 | 13 | 63 | 4 | 18 | 15 | 68 | 6 | 27 | 51 | 18 | |
Table IIA give the results by problem for the Apprentice Schools in the rectangle test ( Set II)
( 289)
| Problem 1 | Problem 2 | Problem 3 | Problem 4 | Total % | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Gr. | No. | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt. | Pr. |
| HS | 19 | 18 | 95 | - | - | 16 | 85 | 3 | 30 | 16 | 84 | - | - | 10 | 52 | 2 | 20 | 70 | 7 |
| 8 | 103 | 96 | 94 | - | - | 67 | 65 | 18 | 18 | 54 | 52 | 9 | 9 | 37 | 36 | 16 | 16 | 62 | 12 |
| 7 | 36 | 33 | 87 | - | - | 17 | 43 | 7 | 18 | 14 | 41 | 3 | 11 | 8 | 18 | 4 | 11 | 48 | 9 |
| 6 | 19 | 14 | 74 | - | - | 9 | 47 | 3 | 16 | 4 | 21 | - | - | - | - | - | - | 36 | 6 |
| Gr. | No. | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W. | N. Att |
| HS | 19 | 1 | 5 | - | - | - | - | -- | - | 3 | 16 | - | - | 7 | 39 | - | - | 15 | - |
| 8 | 103 | 3 | 3 | 1 | 1 | 17 | 17 | 11 | 1 | 35 | 35 | 5 | 5 | 46 | 45 | 3 | 3 | 25 | 3 |
| 7 | 36 | 3 | 13 | - | - | 12 | 37 | -- | - | 14 | 45 | 5 | 10 | 23 | 69 | 1 | 2 | 41 | 3 |
| 6 | 19 | 3 | 15 | 1 | 5 | 6 | 32 | 15 | 5 | 11 | 59 | 4 | 20 | 17 | 90 | 2 | 10 | 49 | 10 |
Table III gives the results by problem for the boys out of school in the fundamentals (Set I).
( 290)
| Problem 1 | Problem 2 | Problem 3 | Problem 4 | Total % | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Gr. | No. | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt. | Pr. |
| HS | 20 | 18 | 90 | - | - | 19 | 95 | - | - | 18 | 90 | - | - | 14 | 70 | - | - | 86 | - |
| 8 | 89 | 73 | 74 | - | - | 60 | 61 | - | - | 53 | 54 | - | - | 29 | 29 | - | - | 54 | - |
| 7 | 35 | 19 | 47 | - | - | 14 | 27 | - | - | 7 | 12 | - | - | 4 | 8 | - | - | 23 | - |
| 6 | 16 | 6 | 36 | - | - | 4 | 27 | - | - | 3 | 18 | - | - | 1 | 7 | - | - | 22 | - |
| Gr. | No. | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W. | N. Att |
| HS | 20 | 20 | 10 | - | - | 1 | 5 | - | - | 2 | 10 | - | - | 5 | 25 | 1 | 5 | 13 | 2 |
| 8 | 98 | 19 | 19 | 6 | 7 | 33 | 33 | 6 | 7 | 28 | 25 | 17 | 18 | 46 | 48 | 23 | 24 | 33 | 14 |
| 7 | 35 | 13 | 40 | 3 | 13 | 17 | 55 | 5 | 22 | 16 | 45 | 12 | 42 | 17 | 47 | 14 | 46 | 47 | 31 |
| 6 | 16 | 8 | 51 | 2 | 13 | 9 | 55 | 3 | 18 | 4 | 24 | 9 | 58 | 6 | 35 | 9 | 58 | 41 | 37 |
The results by problem for the boys out of school, rectangle test (Set II)
( 291)
| Problem 1 | Problem 2 | Problem 3 | Problem 4 | Total % | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Gr. | No. | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt. | Pr. |
| 5 | 18 | 8 | 44 | 4 | 22 | 10 | 56 | 1 | 6 | 6 | 33 | 1 | 6 | 4 | 22 | 3 | 17 | 39 | 14 |
| 4 | 9 | 2 | 22 | - | - | 2 | 22 | - | - | 2 | 22 | - | - | 2 | 22 | - | - | 22 | - |
| 3 | 1 | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - |
| Gr. | No. | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W. | N. Att |
| 5 | 19 | 4 | 22 | 2 | 11 | 4 | 22 | 3 | 17 | 8 | 44 | 3 | 17 | 8 | 44 | 4 | 22 | 44 | 17 |
| 4 | 9 | 5 | 56 | 2 | 22 | 5 | 56 | 2 | 22 | 2 | 22 | 5 | 56 | 1 | 11 | 6 | 67 | 36 | 44 |
| 3 | 1 | - | -- | 1 | 100 | - | - | 1 | 100 | - | - | 1 | 100 | - | - | 1 | 100 | - | 100 |
The results by problem for the boys out of school who fell below the sixth grade in the fundamentals. Attention has already been called to the fact that this is an easier test than that given the other boys
( 292)
| Problem 1 | Problem 2 | Problem 3 | Problem 4 | Total % | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Gr. | No. | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt | % | Pr | % | Rt. | % | Pr. | % | Rt. | Pr. |
| 5 | 17 | 7 | 40 | - | - | 3 | 18 | - | - | 1 | 6 | - | - | - | - | - | - | 16 | - |
| 4 | 9 | 3 | 33 | - | - | 1 | 11 | - | - | - | - | - | - | - | - | - | - | 11 | - |
| 3 | 1 | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - | - |
| Gr. | No. | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W | % | N. Att | % | W. | N. Att |
| 5 | 17 | 7 | 41 | 3 | 18 | 9 | 53 | 5 | 29 | 5 | 29 | 11 | 65 | 6 | 35 | 11 | 65 | 40 | 44 |
| 4 | 9 | 6 | 67 | - | - | 2 | 22 | 6 | 67 | 1 | 11 | 8 | 89 | - | - | 1 | 100 | 25 | 64 |
| 3 | 1 | - | - | 1 | 100 | - | - | 1 | 100 | - | - | 1 | 100 | - | - | 1 | 100 | - | 100 |
(293)
| Grade | No. | Rt. % | Pr. % | W. % | Not Att. % | |
|---|---|---|---|---|---|---|
| In the Apprentice Schools | HS | 6 | 69 | 4 | - | - |
| 8 | 82 | 88 | 7 | 4 | - | |
| 7 | 33 | 76 | 9 | 14 | 1 | |
| 6 | 21 | 66 | 11 | 14 | 10 | |
| In the night schools | 8 | 111 | 76 | 9 | 16 | 1 |
| 7 | 111 | 56 | 11 | 27 | 6 | |
| 6 | 86 | 36 | 6 | 47 | 13 | |
| Boys out of School | HS | 19 | 79 | 7 | 15 | - |
| 8 | 103 | 62 | 12 | 25 | 3 | |
| 7 | 36 | 48 | 9 | 41 | 3 | |
| 6 | 19 | 36 | 6 | 49 | 10 |
Table V shows the location of the boys, the grade, the number of boys, the per cent of problems worked correctly by each grade, etc., in the test on fundamentals for all three groups.
| Grade | No. | Rt. % | Pr. % | W. % | Not Att. % | |
|---|---|---|---|---|---|---|
| In the Apprentice Schools | HS | 6 | 92 | - | 8 | - |
| 8 | 81 | 86 | - | 11 | 3 | |
| 7 | 35 | 62 | - | 26 | 13 | |
| 6 | 22 | 31 | - | 51 | 18 | |
| In the night schools | 8 | 100 | 60 | - | 30 | 8 |
| 7 | 98 | 42 | - | 38 | 22 | |
| 6 | 72 | 34 | - | 37 | 29 | |
| Boys out of School | HS | 20 | 86 | - | 13 | 2 |
| 8 | 98 | 54 | - | 33 | 14 | |
| 7 | 36 | 23 | - | 47 | 31 | |
| 6 | 16 | 22 | - | 41 | 37 |
Table V-A is made up the same as Table V, but shows the grade and group standing in the rectangle test.
| Location | Grade | No. | Rt. % | Pr. % | W. % | Not Att. % |
|---|---|---|---|---|---|---|
| Apprentice Schools | HS | 25 | 88 | 6 | 8 | - |
| Night School | 8 | 296 | 75 | 9 | 15 | 1 |
| Boys out of school | 7 | 180 | 60 | 10 | 27 | 3 |
| 6 | 126 | 46 | 8 | 37 | 11 |
(293)
Table VI is based on Table V and shows the comparative standing of the different grades, rather than the group comparison. To illustrate, the whole number of boys of all three groups of eighth-grade standing have been added together and their averages compared with the total averages of the sixth and seventh grades.
| Location | Grade | No. | Rt. % | Pr. % | W. % | Not Att. % |
|---|---|---|---|---|---|---|
| Apprentice Schools | HS | 26 | 89 | - | 11 | 7 |
| Night School | 8 | 279 | 67 | - | 25 | 8 |
| Boys out of school | 7 | 168 | 42 | - | 37 | 22 |
| 6 | 110 | 29 | - | 43 | 28 |
Table VI-A is based on Table V-A, and is similar to Table VI, except that it deals with the rectangle problems while Table VI deals with the fundamentals.