Space, Time and Deity
1: Space-Time
Chapter 1: Physical Space-Time
Samuel Alexander
Table of Contents | Next | Previous
Extension and duration
IT is not, I believe, too much to say that all the vital I problems of philosophy depend for their solution on the solution of the problem what Space and Time [1] are and more particularly how they are related to each other. We are to treat it empirically, describing Space and Time and analysing them and considering their connection, if any, as we do with other realities. We do not ask whether they are real in their own right or not, but assume their reality, and ask of what sort this reality is. Kant believed them to be empirically real but contributed to experience by the mind, unlike the varying qualities of things which were contributed to experience from things in themselves. Other philosophers have turned to the alleged contradictions in Space and Time, and while assigning to them their due reality as appearance have denied that they are ultimately real, and have maintained that the whole or ultimate reality is spaceless and timeless. Events which in our experience appear in time, that is, are laid out in succession, lose that character in the absorbing whole. This depreciation of Time in particular is a widely spread sentiment among thinking men. When the dying Pompilia in Browning's poem wishes to assure her priest-lover of their true union hereafter, she
(36) sends him the message, " So let him wait God's instant men call years." In a famous passage Kant, speaking of our need of immortality in order to approximate to perfect virtue in an infinite progress, says, " The infinite being for whom the condition of time does not exist sees what for us is an endless series, as a whole in which conformity with the moral law is attained ; and the holiness which his command inexorably requires is present at once in a single intellectual perception on his part of the existence of rational beings." Neither the poet nor the philosopher means merely that what is years to us is a moment to God, in the same way as a moment to a man may be hours to a fly with his microscopic measures of duration. A person might well be content to be an idealist in philosophy in order to have the right of saying these noble things.[2] But all these questions arise not before but after the empirical inquiry into the nature of Space and Time, and this inquiry should answer them directly or indirectly in its course or in its outcome. At the present moment the special question of the exact relation of Time to Space has been forced into the front, because Time has recently come into its full rights, in science through the mathematical physicists, in philosophy also through Prof. Bergson, who finds in Time conceived as dur�e, in distinction from Time as measured by the clock, the animating principle of the universe. Unfortunately his conception of the relation of Space to Time is at once the most important and difficult doctrine of his philosophy and the most obscure. But one welcome consequence of
(37)his work is that it imposes on philosophy the duty of considering, like the mathematicians in their way, what exactly Space and Time are in their relation to one another.
Space and Time as experienced
Space and Time as presented in ordinary experience are what are commonly known as extension and duration, entities (let us say provisionally) or forms of existence, in which bodies occupy places, and events occur at times or moments, these events being either external or mental. We shall deal first with physical Space and Time, leaving mental occurrences to a later stage. Now in order to examine empirically what Space and Time are, it is necessary to consider them by themselves, in abstraction from the bodies and events that occupy them, and this offers great difficulty and may seem to some illegitimate. The difficulty is partly derived from our practical habits, for we are not accustomed to think about Space and Time themselves, but about the things contained in them. But it also has a theoretical basis. For we have not any sense-organ for Space or Time ; we only apprehend them in and through our sensible apprehension of their filling ; by what mode of our apprehension we shall inquire later. I shall call it intuition. It is only by analytic attention that we can think of them for themselves. This leads to two alternative or partially alternative beliefs. Sometimes it is thought that spatiality and temporal character are but properties of sensible things. Extension (to confine ourselves for the moment to this) belongs to colours and touches. In psychology this consideration has brought into authority the doctrine that our sensations, some or all of them have a certain bigness or extensity just as they have quality or intensity. The other alternative is to declare Space and Time to consist of relations between things or entities, these entities with their qualities coming first, and Space and Time are then respectively the order of coexistence and succession of entities. This is the relational doctrine of Space and Time, and it will come up for discussion in its place, where we shall have to ask how far it is justified and whether relations of space and time (whatever they
(38) are) are relations as the doctrine suggests between things or events, or relations between places or times themselves.
The relational view of Space and Time
At first this relational view seems imperative. The ' ordinary mind, impressed with things and events, naively thinks of Space and Time as if they were a sort of receptacle or framework in which things and events are found. The helplessness of such a belief, which makes the connection of things with their space almost accidental, drives us into the relational view. But whatever we may learn later about this relational view, which is of course a legitimate and workable one, it seems clearly not to represent our direct experience of Space or Time. For bodies are not only in relations of space to one another, but they themselves occupy spaces and have shapes ; and though we may regard these in turn as relations between the parts of the bodies, this is surely a theory about them and not a description of what the shapes of things look and feel like. They look and feel like extensions. But in fact the relational view is not the only permissible hypothesis. Another hypothesis as to the connection between things or events and the Space and Time they occupy places in is that Space and Time are not merely the order of their coexistence or succession, but are, as it were, the stuff or matrix (or matrices) out of which things or events are made, the medium in which they are precipitated and crystallised ; that the finites are in some sense complexes of space and time. In the language familiar from the seventeenth-century philosophy, things and events are modes' of these 'substances,' extension and duration. In the same way instead of supposing that extension is a partial character of a colour or a touch, we may suppose colour to be a character of the extension, that what we see is not extended colour but coloured extension. We may even think it possible, as has already been suggested, that although we on our level of existence can see extension only through colour, extension itself may be an `experience' on a lower stage of finite existence. A world is capable of contemplation by us (though only through our thinking or analytical attention, and though we can only apprehend Space and
(39) Time through the special senses), which is anterior to qualities and contains nothing else but Space and Time.
These, however, are speculations for the future. But enough is said to show that to consider Space and Time by themselves, abstract and difficult as it is, is not an illegitimate abstraction, but is in fact nothing but the consideration of things and events in their simplest and most elementary character. The reproaches which have been urged against Kant because he said that you may think away material bodies in Space but you cannot think away Space have no justification.[3] Difficult indeed the process is, and in practice I am accustomed in thinking of Space and Time by themselves to keep constantly pictures of material things and events before my mind and then forget their richness of colours and smells and other qualities ; and I recommend this practice to my readers.
The empirical characters of Space and Time
Physical extension then is presented to us in experience as something within which bodies are placed and move, which contains distinguishable parts but is continuous, so that the parts are not presented as having a separate existence, and which is infinite. Ultimately when we introduce intellectual construction we may distinguish points within Space which again are not independent but continuous. The parts of Space are experienced as coexistent. In like manner Time or duration is experienced as a duration of the successive ; it is continuous, so that its distinguishable parts are not isolated but connected ; in
(40) the end it may be distinguished into moments or instants with the help of intellectual construction, and its parts are successive, and, like Space, it is infinite.
The continuity and infinitude of Space and Time thus spoken of as presented in experience are crude, original characters [4] of them. They do not in themselves imply, though still less do they deny, a theory of the nature of a continuum or an infinite, such as is current in the mathematics of the day. There is something in Space and Time of the nature of uninterruptedness which can be described by no other word than continuity ; and something which is described by the word infinity. Mathematical theories of them are arrived at in the effort to render these crude characters into terms of thought, and they come to crown a precedent reflection which is already contained in ordinary experience of Space and Time.
Spaces and times are apprehended in the first instance just as other things are, if not by sense, at any rate through sense. But sense carries us but a little way in this experience. Only finite spaces and times are presented through sense. But even so our senses give us such evidence as they can of these original characters. For no finite space or time is experienced without a surrounding space or time into which it sensibly flows. And every finite time or space is sensibly continuous or uninterrupted ; it is not an aggregate of parts, but something in which parts can be distinguished as fragments of the whole.
Our further ordinary experience of Space and Time involves the recognition of elements given to us in thought. When we proceed to speak of Space and Time as continuous wholes and distinguishable into points or instants, we are going beyond what we learn through sense and employing ideas, or what are sometimes called intellectual constructions, and are employing also thoughts in the special and proper sense of concepts. Nor is there any reason, supposing the ideas to he well chosen, why we should not do so. For the simplest objects of experience are full of our ideas. A thing of a certain sensible colour
(41) and shape is seen as a man. Half the object is ideal, due to our interpretation of what we see. What we perceive is the object which we sense as supplemented by what we image or think. Space and Time are only in the like case with other experienced things, and to apprehend them we need to use imagination and conception. I can see and touch only limited spaces. But 1 discover that one space is continuous with another, or is included within a larger space, and I can think of a very large space, such as a country two hundred miles square, partly by imagination but largely by conceptions founded on experience of the plan of construction of one space out of smaller ones, and on exact measures of length. We take a sensible space and elaborate and extend it by ideas and concepts. It is still plainer that infinite Space is apprehensible only with the help of thought. Similarly there are no perceptible points or instants, but only durations and extents. But we discover in experience that an extent or a duration admits division continually. Space and Time are so constituted. Accordingly we can construct the idea of a point or instant in a way the reverse of that by which we construct an infinite Space or Time. We start with a finite extent or duration, we imagine it divided, and then we interpret this imagination by the concept that there is no end to the division. A point is thus something which, founded on apprehended reality, is constructed by an act of analytical imagination, which involves also besides the image or idea of a point, the concept of point as the element out of an infinitude of which an extent is made. Such an intellectual construction, or construct, is legitimate, provided at least we make no assumption that the point or the instant can be isolated from other points or instants. By using the constructive idea of point or instant we do not falsify the experienced object, Space or Time, but dissect it into its elements, following the plan of its construction. We must not imagine that the elements are unreal because they are ideal constructions, as the word construction is apt to suggest, any more than we must imagine that a man's back is unreal because I do not see it but only imagine it or have it in idea. For sense
(42)has no monopoly of reality. We reach reality by all our powers. All we have to be sure of is that we use them rightly so that the whole, by whatever powers of ours it is apprehended, shall be itself and self-consistent. When we come to the mathematical treatment of Space and Time we shall return to these intellectual constructions.
Infinity and continuity as comprehended
The infinitude of Space or Time is another of their experienced features and like their continuity is a percept extended by thought. It is indeed the other side of their continuity. It expresses not their uninterruptedness but their single wholeness. And, as with continuity, our thinking discovers but does not make, it only finds, an element in Space which is not discoverable by unaided sense. The sensible or perceptual datum is that each finite space is part of a wider one. The infinite Space is the perceptual datum as qualified by the introduction of this conceptual element. The something or other which we feel to be the larger space of which a finite space is a fragment becomes extended into totality. Thus the infinity of Space does not merely mean that we never can reach the end of it however far we go, though it implies that as a consequence. That would be to describe Space in terms of our infirmity. But we are not concerned with our ways of thinking Space but with Space itself. The infinite Space is thus the positive object of which the finitude of any given portion, apprehended as finite, is the limitation. Infinite Space is positive ; finite space is negative. The infinite is not what is not finite, but the finite is what is not infinite. In this sense Space (or Time) is presented as an infinite thing which is prior to every finite piece of it. " How can finite grasp infinity ? " asks Dryden. He had already been answered by Descartes that however difficult to comprehend, the Infinite is known or apprehended directly and before the finite things which are easier to comprehend. It is thus that infinite Space is given to us in experience.
To comprehend it, reflective (mathematical or philosophical) thought is needed which does not merely embody a formulation of the surface aspect of space- or
(43) time experience, (for that is all the thought that is needed to be aware of infinite Space, so patently is its infinitude displayed), but analyses and probes. What philosophers have adumbrated in this regard, the mathematicians have made luminously clear. Space is infinite because it is self-contained. Choose any selection of its parts according to some law of selection and you find that that selection is itself infinite and contained within the original. It is thus that the sphere or the circle have been used as symbols of a totality because they return with revolution into themselves. An infinite class is defined by mathematicians as one to which a class can be found corresponding, one to one, to the original class and yet a part only of the original class. The series of integral numbers is infinite, not because it has no end,— a mere mental or subjective criterion,—but for that reason. Double, for instance, all the numbers of the series t, 2, 3, etc. The series 2, 4, 6, etc., corresponds to the whole series one to one, but falls wholly within it as a part of it. In this case on account of the infinity of the derived series we cannot say that the whole is greater than its part. Whereas if we take a finite series, t, 2, 3, 4, the doubles of these numbers fall some of them outside the original series, and there is no operation we can perform on them which will yield a different result. Space and Time are infinite in this comprehensible and again perfectly empirical sense.
In like manner, reflective thought attempts to comprehend the given apprehended feature of continuity of Space or Time (or any other continuum). But for convenience I defer the few remarks I can make upon this difficult matter to a later page.[5] It is enough to say that, in a continuum, between any two members another can be found.
Space and Time then are presented to us as infinite and continuous wholes of parts. I shall call these parts points and instants, availing myself of the conceptual description of them, and meaning by their connectedness or continuity at any rate that between any two points or
(44) instants another can be found. To me, subject to what may be said hereafter, this is a way of saying that the points and instants are not isolated. But if any reader jibs, let him substitute lengths and durations ; he will find that nothing is said in what follows except what follows equally from the notion of parts.
The interdependence of Space and Time. (1) Time and Space.
Other features will declare themselves as we proceed, some obvious, some less so. But they will be found to require for their understanding the understanding of how Space and Time are related to each other. These are often thought, perhaps commonly, to be independent and separate (whether treated as entities as here or as systems of relations). But a little reflective consideration is sufficient to show that they are interdependent, so that there neither is Space without Time nor Time without Space ; any more than life exists without a body or a body which can function as a living body exists without life ; that Space is in its very nature temporal and Time spatial. The most important requirement for this analysis is to realise vividly the nature of Time as empirically given as a succession within duration. We are, as it were, to think ourselves into Time. I call this taking Time seriously. Our guides of the seventeenth century desert us here. Besides the infinite, two things entranced their intellects. One was Space or extension ; the other was Mind. But entranced by mind or thought, they neglected Time. Perhaps it is Mr. Bergson in our day who has been the first philosopher to take Time seriously.
Empirically Time is a continuous duration, but it is also empirically successive. Physical Time is a succession from earlier to later. As Mr. Russell points out,[6] the succession from past through present to future belongs properly to mental or psychical time. But so long as we take care to introduce no illegitimate assumption we may conveniently speak of past, present, and future in physical Time itself, the present being a moment of physical Time fixed by relation to an observing mind and
(45) forming the boundary or section or cut between earlier and later, which then may be called past and future. In a manner, earlier and later are, as it were, the past and future of physical Time itself. I shall therefore use liberty of phrase in this matter. Now if Time existed in complete independence and of its own right there could be no continuity in it. For the essence of Time in its purely temporal character is that the past or the earlier is over before the later or present. The past instant is no longer present, but is dead and gone. Time's successiveness is that which is characteristic of it as empirically experienced, in distinction, say, from Space, which also is continuous. This is the plain conclusion from taking Time seriously as a succession. If it were nothing more than bare Time it would consist of perishing instants. Instead of a continuous Time, there would be nothing more than an instant, a now, which was perpetually being renewed. But Time would then be for itself and for an observer a mere now, and would contain neither earlier nor later. And thus in virtue of its successiveness it would not only not be continuous but would cease even to be for itself successive. If we could suppose an observer and events occurring in time, that observer could distinguish the two ' nows ' by the different qualities of the events occurring in them. But not even he could be aware that the two nows ' were continuous, not even with the help of memory. For memory cannot tell us that events were connected which have never been together.
Descartes did, in fact, declare the world to be perpetually re-created. For him the idea of a Creator presented no problem or difficulty, and with his imperfect grasp of the real nature of Time the step he took was inevitable and imperative. For us the case is not the same, even if re-creation at each moment by a Creator left no difficulty unsolved. But in any case the universe at the stage of simplicity represented by mere Time arid Space has no place for so complex an idea as creation, still less for that of a supreme Creator. Time and Space are on our hypothesis the simplest characters of the world, and the idea of a Creator lies miles in front.
(46)
Thus the mere temporality of Time, its successiveness, leaves no place for its continuity or togetherness and seems to be contradictory to its continuity. Yet the two are found together in Time as we experience it. If, therefore, the past instant is not to be lost as it otherwise would be, or rather since this is not the case in fact, there needs must be some continuum other than Time which can secure and sustain the togetherness of past and present, of earlier and later. (' Togetherness ' here is used obviously to mean merely connection and not as in ordinary usage contemporaneity.) This other form of being is Space ; that is, Space supplies us with the second continuum needed to save Time from being a mere now.'
The same conclusion follows if, for instants, we substitute durations. The earlier duration, if Time stood by itself, would not be continuous with the later. We should but have a duration, a particle of time, perpetually re-created. There would then, moreover, be the additional problem of how a particle of duration could be temporal if it did not itself exhibit differences of before and after.
It was not open to us to say that since the successiveness of Time and its continuity are contradictory Time is therefore not real but only appearance. Time is an object given to us empirically. We had thus to ask whether this Time is independent of Space, as it appeared to be. With the necessity of Space to the existence of Time the contradiction is removed. It may, in fact, be suggested that the reason why Time and Space are believed to be contradictory in themselves is that the Time and Space in question are not really Time or Space, as they are experienced. Time is considered apart from Space and Space from Time.
The only other way of evading the force of this analysis of facts is on the relational view of Time where an instant is defined by events in relation. But this method is contrary to our hypothesis, and it would be out of place to consider the alternative here.
One word to anticipate misunderstanding. I said that to supply continuity to the successive there must
(47) be a non-successive continuum, simulating by the word ' must ' the deductive form. But I am making no attempt to prove the existence of Space. There is no room for must' in philosophy or in science, but only for facts and the implications of them. I might have said simply the continuity is, as a matter of fact, supplied by the connection of Time with the other continuum Space. The apparent demonstration ' was a piece of analysis of an entity given in experience.
(2) Space and Time
But if Time cannot be what it is without indissoluble relation to Space, neither can Space be except through indissoluble connection with Time. For Space taken by itself in its distinctive character of a whole of coexistence has no distinction of parts. As Time in so far as it was temporal became a mere now,' so Space so far as merely spatial becomes a blank. It would be without distinguishable elements. But a continuum without elements is not a continuum at all. If Space were without elements it would be open to the difficulties urged with so much force against Spinoza's conception of the infinite Substance or of its attributes : that it swallowed or absorbed everything which might be said to be contained in it, but left no means for the existence within it of the multiplicity of things or indeed of anything. Thus the empirical continuity or totalness of Space turns out to be incompatible with the other empirical feature of Space, that it contains distinctness of parts. That distinctness is not supplied by the characteristic altogetherness of Space. There must therefore be some form of existence, some entity not itself spatial which distinguishes and separates the parts of Space. This other form of existence is Time. Or in order once more to avoid the appearance of an attempt to demonstrate the reasons for the universe, let us say that Time is discovered to supply the element in Space without which Space would be a blank.
Thus Space and Time depend each upon the other, but for different reasons. But in each case the ultimate reason of the presence of the other is found in the continuity which in fact belongs to each of them as we
(48) find them in fact. Without Space there would be no connection in Time. Without Time there would be no points to connect. It is the two different aspects of continuity which compel us in turn to see that each of the two, Space and Time, is vital to the existence of the other.
It follows that there is no instant of time without a position in space and no point of space without an instant of time. I shall say that a point occurs at an instant and that an instant occupies a point. There are no such things as points or instants by themselves. There are only point-instants or pure events.[7] In like manner there is no mere Space or mere Time but only Space-Time or Time-Space. Space and Time by themselves are abstractions from Space-Time, and if they are taken to exist in their own right without the tacit assumption of the other they are illegitimate abstractions of the sort which Berkeley censured. How they come to be distinguished apart from one another and on what terms this is legitimate and useful will appear in due course. But at least they are not merely two concurrent though correlated continua. The real existence is Space-Time, the continuum of point-instants or pure events.
Repetition in Space and Time
The characters which Space and Time present to experience and the relation of the one to the other as founded on those characters are not exhausted by the simple statement that each is necessary to the existence of the other. So far the exposition has gone, their correspondence might be a one to one correspondence, to each instant a point. As a matter of fact it is a one-many correspondence. One instant may and does occupy several points, that is, Time is repeated in Space. In the more familiar but less elementary and more complex language of our experience of things,, and events with their qualities, several such physical' events may occur in different places at the same time. Again Space is repeated in Time ; one point may and does occur
(49) at more than one instant ; or to revert to the familiar language of unspeculative experience, several events at different times may occupy the same place. Time, as it were, returns to its old place at a later instant. These mere empirical facts are sufficient to show that the correspondence of point to instant is not one to one. Now it may be seen that our previous statement of the relation of Space and Time was defective, and besides not making provision for the repetition of Space in Time and Time in Space, would be insufficient even to account fully for the continuity of either Space or Time, either an extended continuum or a successive one.
If the correspondence were unique, neither would Space be able to perform its office of saving the instant from perishing, nor Time its office of saving Space from blankness. For each would in that case be " infected," if I may borrow from Mr. Bradley a picturesque manner of speech, with the character of the other. Consider Time first. If the point corresponded uniquely to the instant it would share the character of the instant, and Space would cease to be the Space we know. The point would lack that element of permanence, that is, independence of its particular instant, in virtue of which it can as it were detain the instant and save it from perishing utterly and being a mere now' without connection with other instants. But the repetition of a point at many instants, its recurrence, secures to the point this capacity ; or if the more demonstrative form of words be preferred, in order that Time should linger Space must recur, a point must be repeated in more than one instant. All that our previous analysis effected was to show that a continuous succession depended on something different in itself from a succession. But just because of its intimacy of relation to Time, this something different must be something more than merely different from Time, and that more which it has is what I shall call, for reasons to be made clearer hereafter, the intrinsic repetition of a point in several instants.
Similarly, if there were unique correspondence, Time would share the character of Space, be infected with
(50) bare blank extendedness, would in fact be mere extension and cease to be the Time we know, which is duration in succession. In order that it should be in its own nature successive and so be able to discriminate points in Space, the instant of Time must be repeated in or occupy more points than one ; that is to say, the occupation by an instant of several points gives structure' to the instant, and thereby enables it to distinguish one point of space from another.[8]
This abstract or elementary relation of Space and Time lies, it will be seen, at the bottom of our experience of empirical substances. They possess temporal permanence and spatial structure : the parts of a substance are always changing. There would be no substance were it not that at any instant of its life several parts were of the same age, and each part of its space could be occupied by different moments of time. If the substance changed all at once and its parts were not repeated in time, or if none of its parts occurred together at the same instant it would not be a substance. What I have done above is to exhibit these same relations in respect of any part of Space and Time themselves. Later on we shall realise the importance of this analysis of permanent structure in things, when we come to speak of categories, a topic which I must not here anticipate.
The three dimensions of Space and the character of one dimensional time.
The relation of Space and Time is, however, still more intimate. So far as we have gone Space might be one-dimensional like Time, but physical Space as presented in experience has three dimensions. Time also, besides being a one-dimensional continuum of duration in succession,[9] has two other features as experienced. It is irreversible in direction, that is, an instant which is before another cannot be after it. In technical phrase the relation of instants is
(51) asymmetrical. Secondly, each instant is between two instants, before the one and after the other ; or, to put the same thing otherwise, the relation of before is transitive, that is, if an instant A is before an instant B, and B before C, A is before C. Now the three dimensions of Space are, considered spatially,[10] independent : position may vary according to each independently of the others. To say that Space is a three-dimensional form of externality is the same thing as to say there are three independent one-dimensional forms of externality. It is not so obvious that the three features of Time, its successiveness, the irreversibility of the succession of two instants, and the transitiveness of before,' or betweenness,' are independent. The second is clearly enough different from the first, there is nothing in the relation of successiveness as such which makes it irreversible. But even if Time is irreversible it is not necessarily uniform in its direction. The movement of Time might be pendular, and a movement might be from A to B and from B to C and both irreversible, but that from B to C might be in the opposite direction in spatial representation. It would not in that case necessarily be true that if A is before B and B before C, A is also before C.
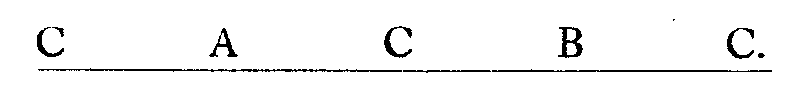
Any of the three relative positions of C is possible, and it could not be said whether A is before or after C—the relation of A to C is indeterminate. In fact, movement in time might be subject to constant reversals of direction by jumps, and any one interval would be irreversible, but the whole Time not uniform in direction, which is necessary to 'betweenness.'
Now the three features enumerated in Space and Time being independent, we might content ourselves with saying that as between spatiality and successive duration there subsists such a connection of interdependence that each new feature in Time is rendered possible by a new dimension of Space and conversely renders it possible. But I do not like to leave the matter in this vague
(52) condition ; and therefore I shall try to make the connection of Time's properties and the dimensions of Space more explicit ; hazardous as the undertaking is. It is essential to bear in mind the one-dimensionality of Time and the independence of the three dimensions of Space of one another. I shall use capital letters to designate instants and small ones to designate points.
Irreversibility of Time and a second dimension of Space
Let us begin with the fact of irreversible, that is, determinate, order in time ; we can see then that a one-dimensional Space would not suffice to secure it. aA and bB are two point-instants. The points a and b which occur at those instants suffice to distinguish the instants as well as making them possible, but not to determine whether A is prior to B or posterior. So far as the points are concerned A might be before or after B in time. For the instant A is repeated in space at say the point al, and if there were only one dimension of Space and we take the line ab as we may to represent the time-dimension as well, there would be nothing to distinguish aA from a1A, which has the same time. But a, might be on the other side of b from a and thus A might be either before or after B.[11] Hence since the order is irreversible it follows that the instant A cannot be repeated in the one-dimensional line ab. For it is clear
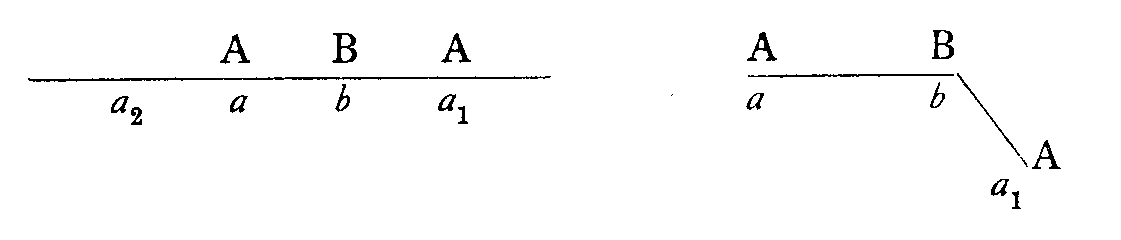
that A cannot be repeated at two points a and a2 both on the same side of b, because in that case their dates
(53) would be different.[12] Now with a second dimension of Space A can be repeated outside the line ab, and since this second dimension is independent of the first, the possible contradiction is removed. The point a is before b so far as one dimension is concerned, and the point a1 before b so far as the other is concerned. The second dimension is accordingly not only necessary but sufficient. Thus if succession is irreversible there needs more than one dimension of Space.
Conversely if Space has two dimensions succession is irreversible. Let XB now represent the one-dimensional time-line, X and B occupying the points x and b; and let a be a point outside xb occurring at the instant A, which is before B.[13] Now every point is repeated in time and a therefore occurs at some other instant A1 which may
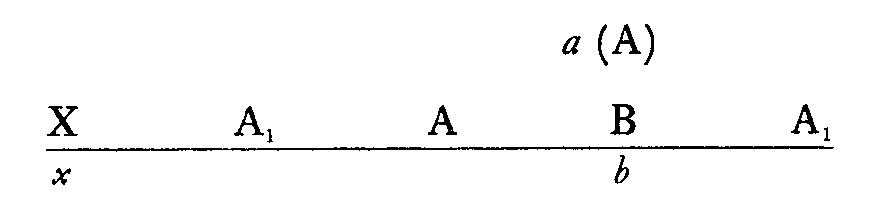
be represented on the time-line on either side of B from X. But unless the order in time is irreversible there is nothing to distinguish A from A, since they both occupy the same point, though the two point-instants are different. Al might be before B or after it, so far as Space is concerned.
(54) There must he something therefore in Time to distinguish the two point-instants aA and aA1. This is secured by the irreversibility of the time-order. A1 is after B, or if before B at a different date from A.
Note in passing and by way of introduction to what follows that nothing has been said as to whether A is or is not before A1. All we need is that A1 if after B shall not also be before B, and irreversibility secures this. It is therefore not only necessary for distinguishing aA from aA1, but it is sufficient for the purpose.
Betweenness of Time and a third dimension of Space.
The third correspondence, that of the betweenness of Time to a still further independent dimension of Space, is more difficult to establish. Suppose, in the first place, that succession possesses betweenness or is a transitive relation. Then it may be seen that two dimensions of Space are insufficient. Let there be a movement in such Space from a to b and from b to c, so that the instant A of a is before B and B before C. Since Time is one-dimensional we may represent times, as was done before, on the line ab ; and on this C also may be represented. Now the
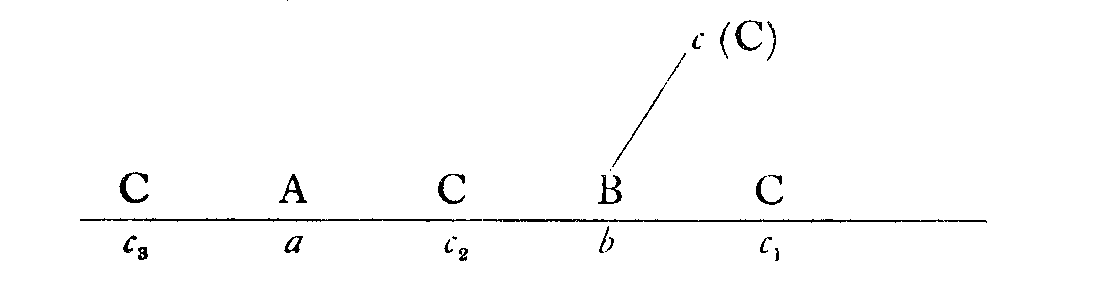
instant C of the point c is repeated in space, and not in the line bc, and since ab represents the time-line, we may represent the various repetitions of C in space by points on the line ab. But C is then represented ambiguously by any one of the three points c1, c2 or c3. For whichever of the points repeats the time C, the postulate of irreversible succession is satisfied. If C is at c3 A is before B, but B may still be before C, for Time may change its direction within the line ab, but still C will be after B. So, for example, there is irreversible succession in the swings of a pendulum, but as represented by the excursion the time changes its direction. There is irreversible order in each of the two times AB, BC ; but there is no betweenness unless C falls at c1. There is thus no guarantee
(55)of betweenness of Time if the repetition of C be confined to two-dimensional Space. But this is possible if C be repeated outside the plane abc. Moreover, since the third dimension is a new dimension and independent of the other two, the necessary condition is also sufficient. For if the relation of A, B, and C is transitive, we must represent the priority of B to the instant C in the new independent dimension by the same spatial convention as we represent priority on the line ab, that is, C occupies c1 and not c2 or c3.
Conversely, if Space is three-dimensional Time is transitive, there is not merely irreversibility of direction in Time but uniformity of direction. Let the line XB represent the time-line, these being the instants of x and b. B is before C, which is the instant of the point c, and there is a point a
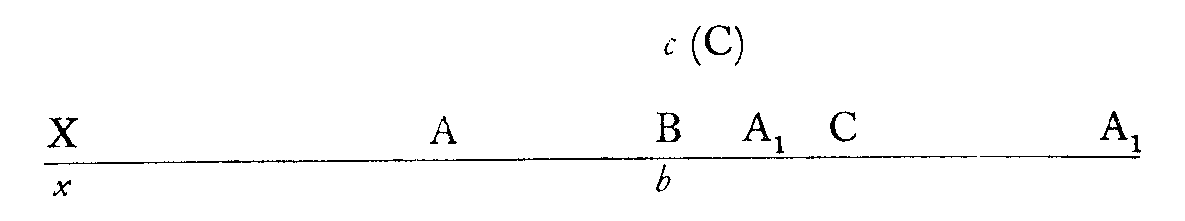
outside the plane of the paper whose time A is before B. But a is repeated in time at the instant A1, which may be represented on the time-line on either side of C from B. Whether a occurs at A or A1 there is irreversibility of succession. But there is nothing so far as Space is concerned to distinguish A and Al since they occupy the same point a, although the point-instants aA and aA1 are different point-instants. Hence if we only know that a occurs at an instant A before B, we cannot tell where that instant is to be represented on the one-dimensional time-line, unless there is some property of Time which is different from irreversibility. On the other hand, betweenness secures the definite place of a upon the timeline, and is thus not only necessary but sufficient if there is to be a third and independent dimension of Space. As in the earlier case of the proof of determinate order as necessary with a second dimension, the reasoning -depends on securing the unambiguous distinction of point-instants. For no point-instant can be repeated, while both the point and the instant may.
(56)
It follows as a matter of course that since every instant is connected with other instants continuously, in definite order and in the transitive relation, every point is related to other points in three dimensions, and is therefore voluminous. The physical point is in fact the limit of a volume.
Order
It is difficult in discussing the elementary relations of Space and Time to avoid questions which belong to a later stage of our inquiry. One such question will have at once arisen in the minds of some. Irreversibility and betweenness have been on the faith of experience attributed to Time, and tridimensionality to Space. But why, it may be asked, is not Space credited with irreversibility of order and betweenness ? Certainly if a is to the right of b, b is not to the right of a ; and if a is to the right of b and b to the right of c, b is between a and c. Has not the character of Time been represented by a line in Space so as to imply these characters of points of Space ? The truth is that these are characters of order, and are so because they belong intrinsically to Time, and they .belong to order because all order presupposes Time. Points in space are ordered in virtue of their time - character. This does not mean that they assume an order through our act in arranging them or selecting them by a process which takes time. Our procedure in dealing with positions in space or time has nothing to do with these positions themselves ; at least it is the hypothesis of the present inquiry that the mind is merely contemplating what it finds in Space and Time. Our proposition means that positions in space are really ordered themselves, but that they are so ordered in virtue of the time-character which is essential to them. Merely as points, as positions in space, they do not possess order, any more than instants merely as temporal possess position in time—supposing that it is possible to think of points apart from their time or instants apart from their space, which we have seen it is not. Order arises out of the temporal character of positions in space ; and it is this fundamental or elementary order which is presupposed in any order of more complex or qualitative things which are in any way
(57) ordered, like pebbles in a row, or terms in a progression, or officials in a hierarchy.[14]
A caveat
Let me, at the conclusion of this inquiry (which, be it observed, is entirely non-mathematical) into the precise relation between Space and Time, once more remark that it contains no attempt at a construction of Space and Time, as if we were giving reasons for them and for their experienced features, and in a manner affecting to preside over their creation. Such an attempt would be as foolish as it is unscientific. I have merely attempted to show how the various features of the one depend for their character on those of the other. The reason why Space has three dimensions is that Time is successive, irreversible, and uniform in direction. If we could imagine a Creator who had determined to make Time an asymmetrical transitive succession, he would, to carry out his purpose, have made a Space of three dimensions ; and vice versa if he had determined to make a three-dimensional Space he would, to carry out his purpose, have made Time a transitive asymmetrical succession. This does not mean that one who knows the characters of Time could conclude from them to the three dimensions of Space, but only that he finds on examination that there is an intimate relation between the one and the other, a relation which requires reflective analysis to discover. The word must' or needs' which I have occasionally used means no more than that we are forced to look for something, which we may or may not find. We cannot say that Time implies Space in the sense in which the working of two laws in conjunction implies their resultant. The point is of sufficient general importance to be worth a few remarks even here. Suppose a man thought, I will not say that he could see a reason why there must be Space, but that he could see a reason why if there was to be Space there must be Time or vice versa, he might be asked how in the absence of an experience of Time he would be able to invent the idea of Time. All that he can do is to see that in Space as empirically presented there are features which are those of Time as empirically presented, and that the mutual relation
(58)of Time and Space is so close and ramified that they cannot be considered as separate entities but only as the same entity described in terms of its different elements. Other `must' metaphysics does not recognise—except the must of logical implication, and wherever I have spoken of implication I have been careful to limit it to what the experience with which I have been dealing itself demands, and only so far as it demands it. The undertaking is hazardous and even presumptuous enough (and I cannot feel complete confidence that some error may not have crept in) not to be burdened with the suspicion of pretensions which are foreign to it.
Space-Time: (1) in mathematics;
We have thus by purely analytical or metaphysical and non-mathematical methods applied to a subject-matter presented in experience, arrived at a notion of Space-Time which at least in spirit is not different from the notion of a world in Space and Time which was formulated by mathematical methods by the late H. Minkowski, in 1908. The underlying conception had been used or implied in the memoirs of Messrs. Lorentz and Einstein, which along with Minkowski's memoir laid the basis of the so-called theory of relativity, which is now, I believe, common property amongst certain mathematicians and physicists. In Minkowski's conception the Universe is a system of world- or cosmic points ; it is assumed that at each point-instant (the name[15] is due to Mr. Lorentz, Ortszeit) there exists some perceptible " substance," and the course of such a substantial point is called a cosmic line (world-line, Weltlinie). Space and Time are described as being shadows of the Universe. Only the Universe has self-existence. Every point has four co-ordinates, the time co-ordinate being the fourth. Hence it follows, as Minkowski writes, that geometry with its three dimensions is only a chapter in four-dimensional physics. There are infinite Spaces in the physical world, just as there are infinite planes in our Space. In fact, Space
(59) becomes merely the assemblage of all events which belong to the same moment of Time.
Now I understand the essential spirit of the doctrine to be that Space and Time are not independent of each other but united in the one four-dimensional world, and with this the result of our empirical or metaphysical inquiry is in agreement. But there are some respects in which there is (as I must with becoming misgivings admit) divergence. I take for granted that to think of Time as a fourth dimension in a world in which the other three dimensions are spatial is a legitimate and the only possible way of representing mathematically the nature of the world or Space-Time. But if the empirical analysis is correct, this representation cannot be regarded as other than a means of mathematical manipulation. For it seems to treat Time as an additional dimension, not of course a spatial one, much in the same way as the third spatial dimension is additional to the other two, that is, as a further order in which three-dimensional Spaces are arranged. But the relation between Space and Time which we have found empirically appears to be of a much more intimate kind than is thus suggested. For not only are Space and Time indispensable to one another (as in the conception of Minkowski), but Time with its distinctive features corresponds to the three dimensions of Space, and in a manner of speech Time does with its one-dimensional order cover and embrace the three dimensions of Space, and is not additional to them. To use a violent phrase, it is, spatially, not temporally, voluminous. Metaphysically, (though perhaps mathematically), it is not therefore a fourth dimension in the universe, but repeats the other three. Space, even to be Space, must be temporal.'[16]At a later point[17] I shall propose a non-mathematical formula which seems to me to express this relation with metaphysical propriety, without attempting to question the mathematical
(60) appropriateness of Minkowski's formulation. Beyond this, I call attention only to two other matters. According to the method customary in mathematics, it is taken for granted that there is a substance, electricity or matter or what not (the word substance is used expressly in order to avoid the question), which occupies the point-instant. On our hypothesis, whatever substance there is must be a fragment of the one stuff of Space-Time, and therefore is not to be assumed within the metaphysical account of Space-Time. Secondly, as will be mentioned presently, the definition of Space as the assemblage of events at one instant, which may be derived from Minkowski's doctrine and is formulated so by Mr. Langevin,[18] is connected with the same assumption of material events occurring at a point-instant, and while legitimate in one way, and true, does not tell us the essential character of Space but a consequence of it.
Space-Time: (2) in metaphysicz
Meantime, I may proceed with the metaphysical exposition. We have then to think of Space and Time in much the following way. (a) By themselves each consists of elements or parts which are indistinguishable so long as the elements of the other are excluded. (b) In reality each point of space is determined and distinguished by its instant in time, and each instant of time by its position in space. The elements of the one reality which is Space-Time, and not either Space or Time alone, owe their distinctness in either kind to the complementary element. We have not yet arrived at an examination of the notions of identity and diversity. But using these terms in their common sense, either of the two we may regard as playing the part of identity to the other's part of diversity. It is worth while observing this, because previously Time was shown to supply discrimination in the otherwise blank Space. But Space may equally well he regarded as introducing diversity into time. For without Space Time would be a bare 'now '
(61) always repeated, and there would be no such thing as diversity. But the reality of Space and Time is in Platonic phrase [19] the " substance " which contains the identity and the diversity in one.
Space must thus be regarded as generated in Time, or, if the expression be preferred, by Time. For Time is the source of movement. Space may then be imaged as the trail of Time, so long as it is remembered that there could be no Time without a Space in which its trail is left. It would be inept to say that Time is in its turn the trail of Space, for Space of itself has no movement. The corresponding proposition is that Time as it moves from past through present to future (from earlier to later) is the occupation of a stretch of Space.
Space-Time thus consists of what may be called lines of advance connected into a whole or system in a manner to be described. In a line of advance c b a we have the displacement of the present from c through b to a, so that a becomes present while b becomes past and c still further past. The present means as before the point of reference. In terms of earlier and later, b having been later and c earlier, a becomes later and cb earlier. Now this is the meaning of motion. Points do not of course move in the system of points, but they change their time-coefficient. What we ordinarily call motion of a body is the occupation by that body of points which successively become present, so that at each stage the points traversed have different time-values when the line of motion is taken as a whole. Thus Space-Time is a system of motions, and we might call Space-Time by the name of Motion were it not that motion is in common speech merely the general name for particular motions, whereas Space easily and Time less easily are readily seen to be wholes of which spaces and times are fragments. Hence Descartes could identity Space with matter, and there is nothing astonishing in the hypothesis that Space as qualified with Time is the matrix of all being. But Motion we find it difficult so to represent to ourselves. It seems paradoxical consistently with the ordinary use of
(62) language to speak of a single vast entity Motion, though to do so is to do the same thing as to speak of Space-Time.
Pictorial representation of Space-Time
But the notion that Space is generated in Time in the form of motion is apt to be misinterpreted. We may think of fresh Space as being swept out in Time. We figure advance in Time, the growth of the world, as an advance in column, and it is then easy to go on and treat the present moment as determining a section along this advance, so that Space becomes the arrested events of the present or any one moment. We then have the idea of an infinite spatial present sweeping forward in Time. Space is defined as the assemblage of events at one moment. We shall find that this proposition has under proper conditions a good and important meaning. But both it and the previous proposition that Time sweeps out fresh Space in its advance are open to fatal objections.
First of all, to suppose that Time generates new Space is to neglect the infinity of Time (and indeed of Space). It supposes a part of Space to be generated at the beginning and pushed forward. But a beginning of existence is itself an event in Space-Time which is the system of point-instants or pure events, and it is clear therefore that Space-Time as a whole begins either everywhere or nowhere. Infinity is understood here as explained before in its true sense of self-containedness.
Secondly, the notion that Space is what is occupied by any moment fails to give a true insight into the intrinsic nature of Space. A present which occupies the whole of Space would suffer from the same defect as a mere instant disconnected with other instants. We saw that an instant which was not through Space connected with its past would not be an instant of Time at all, because its past would have perished and it would perish too. The present so described would be all that there was of Time, a now ' perpetually re-created. If it were spread over the whole of Space, it and its Space would need to be re-created at each moment.
We have then to abandon the notion of an advancing
(63) column and of Space as a mere instantaneous section of that advance. We have to think of lines of advance as displacements of the present in relation to past and future over positions in Space. In this way we conceive of growth in Time, or the history of the Universe as a whole, or any part of it, as a continuous redistribution of instants of Time among points of Space. There is no new Space to be generated as Time goes on, but within the whole of Space or the part of it the instants of Time are differently arranged, so that points become different point-instants and instants become also different point-instants. I believe that this very abstract (I mean very simple, yet highly concrete) conception lies, in fact, very near to our common notions of a growing world.
But an abstract conception is difficult to retain without a pictorial and more complex representation, and there are several at hand to replace the misleading image of an advancing column. The simplest way is to imagine a limited space, and motions taking place within it. We may choose a disturbed ant-heap or the less pleasing instance of a rotten cheese seen under the microscope. But a severer and more useful picture is that of a gas in a closed vessel, conceived according to the kinetic theory of gases. The molecules of the gas dash against the sides of the vessel and each other in all manner of lines of advance, whether straight lines or not is for us indifferent. The molecules stand for instants of Time with their dates, some being earlier and some later, in various degrees of remoteness, than the point-instant which is the centre of reference ; some simultaneous with it, that is, possessing the same date. The gas is not considered as it is at any moment but as it exists over a lapse of time. We are not supposing the internal motions to be arrested at a given instant which is taken as the point of reference, which would be to suppose arrested what is intrinsically a movement. For us it is perfectly easy to contemplate the motions of the gas over such a lapse of time, for we have memory to help us and expectation, and we can keep in our minds at once a limited piece of the history of the gas. Subject to this explanation we can revert to our
(64) usual form of speech and say the molecules are some of them present instants, some past, and some future instants, and the incongruousness of future instants disappears, for they are objects of our minds;, that is, objects in expectation, equally with present and past instants. Like all pictures which symbolise a conception the image halts ; and in two respects. First, the instants of identical date are separate molecules, whereas simultaneous instants, as we have seen, are the same instant, and have no temporal but only spatial difference. Here both the molecules and their places are distinct. Secondly, there need not always be a past molecule to take the place of the present' one as the present one moves on to a new point in space, leaving its old place to be past or earlier. There are places in the gas empty of molecules. But in Space-Time there is no place without time. Still the picture conveys fairly well the notion of redistribution of instants among points. In some respects the streaming of protoplasm in a cell would be a more manageable image. Best of all perhaps, and certainly very useful, is the picture of the condition of a growing organism where we find a perpetual alteration or redistribution among the cells of distance from maturity ; some being mature (the present), some moribund, in different stages of senescence, and still others adolescent.
We have now to see how Space is to be thought of more accurately as saturated with Time, that is to say, as the theatre of perpetual movement ; and secondly, in what sense it is true to say that any instant occupies the whole of Space, or that any point occurs in the whole of Time.